Capacitance Formula Comprehensive Guide

In the world of electrical engineering, capacitors are indispensable components that store and release electrical energy. Understanding the formula governing capacitance is essential for designing circuits, analyzing their behavior, and optimizing performance. This article aims to delve into the intricacies of capacitance formula, exploring its definition, calculation methods, and practical applications in electronic circuits.

What is the Formula of Capacitor?

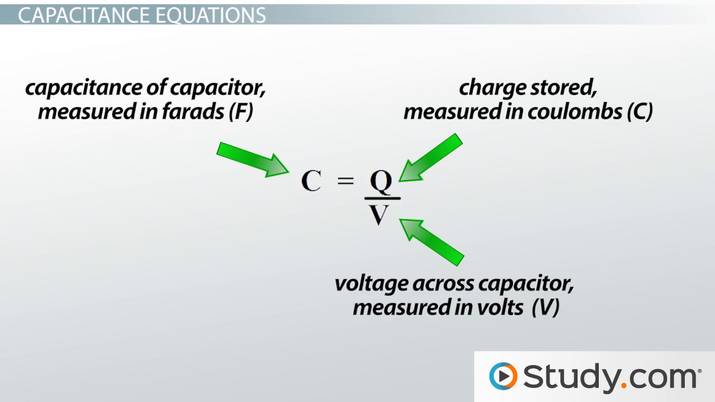
The formula for calculating capacitance (C) of a capacitor is derived from its physical characteristics, specifically its geometry and dielectric properties. Mathematically, capacitance is defined as the ratio of the electric charge (Q) stored on the capacitor's plates to the voltage (V) across the plates. The formula is represented as:

C=Q/V​

Where:

• $C$ is the capacitance measured in Farads (F),
• $Q$ is the electric charge stored on the capacitor plates in Coulombs (C),
• $V$ is the voltage across the capacitor plates in Volts (V).

 Helpful resource: http://labman.phys.utk.edu/phys222core/modules/m2/capacitors.html

How is Capacitance Calculated?

The capacitance of a capacitor depends on its physical properties, primarily the area of the plates, the distance between the plates (dielectric thickness), and the dielectric constant of the material between the plates. The formula for calculating capacitance for a parallel plate capacitor, one of the most common types, is given by:

C=ε⋅A/d​

Where:

• $C$ is the capacitance measured in Farads (F),
• $\epsilon$ is the permittivity of the dielectric material between the plates (in Farads per meter, F/m),
• $A$ is the area of the plates overlapping each other (in square meters, m²),
• $d$ is the distance between the plates (in meters, m).

For capacitors with other geometries, such as cylindrical or spherical capacitors, different formulas may apply based on the specific configurations. Helpful resource: https://study.com/skill/learn/how-to-calculate-the-capacitance-of-a-parallel-plate-capacitor-of-given-its-geometry-explanation.html

How Do You Calculate Capacitance from Voltage and Current?

Capacitance can also be calculated using the relationship between voltage, current, and time during the charging or discharging process of a capacitor. When a capacitor is connected to a voltage source through a resistor, it charges up until it reaches a steady-state voltage determined by the source voltage and the resistor value. The charging process follows an exponential curve, and the capacitance can be calculated using the time constant ($\tau$) of the circuit and the resistance ($R$):

C=Q​/V=I⋅t​/V=I⋅R⋅t/V​

Where:

• $C$ is the capacitance measured in Farads (F),
• $Q$ is the electric charge stored on the capacitor plates in Coulombs (C),
• $V$ is the voltage across the capacitor plates in Volts (V),
• $I$ is the current flowing through the circuit in Amperes (A),
• $t$ is the time taken for the capacitor to charge or discharge (in seconds, s),
• $R$ is the resistance of the circuit in Ohms (Ω).

By measuring the voltage across the capacitor ($V$), the current flowing through the circuit ($I$), and the time taken for the charging or discharging process ($t$), the capacitance ($C$) can be determined using the above formula. Helpful resource: https://phys.libretexts.org

In conclusion, understanding the capacitance formula is essential for analyzing and designing circuits involving capacitors. Whether calculating capacitance based on physical parameters or determining it from voltage, current, and time measurements, mastering the capacitance formula is crucial for engineers and enthusiasts alike in their pursuit of creating efficient and reliable electronic systems.

 

---

 

Facts Checked by Hugh Johnson
Hugh Johnson stands tall in the realms of both the Integrated Circuits (ic Chips) industry and the electronic parts industry, earning acclaim as an esteemed expert in these fields. With an extensive background steeped in semiconductor technology, Hugh's expertise transcends chip design and fabrication, encompassing a profound understanding of electronic components' intricate functionalities and applications. His seasoned knowledge spans diverse facets, from microchip architecture and fabrication techniques to the broader landscape of electronic parts utilized across industries.